Accessible Algorithms For Addition - Addition Worksheet
ADVERTISEMENT
Level 2
(continued)
Accessible Algorithms for Addition
Introduce the accessible algorithms presented below when
students learn about adding with regrouping. Support students’
use of these algorithms whenever they are working on multi-digit
addition or decimal addition.
New Groups Below Method
Use with Houghton Mifflin Math, Ch 3, Lessons 5, 8; Ch 22, Lesson 4.
This algorithm helps students see the relationships between
single-digit addition and the regrouping process that takes place
in multi-digit addition. Instead of using the common algorithm
of recording the new ten or hundred above the tens or hundreds
digits in the equation, students learn to record the new ten or
hundred below the tens or hundreds digits.
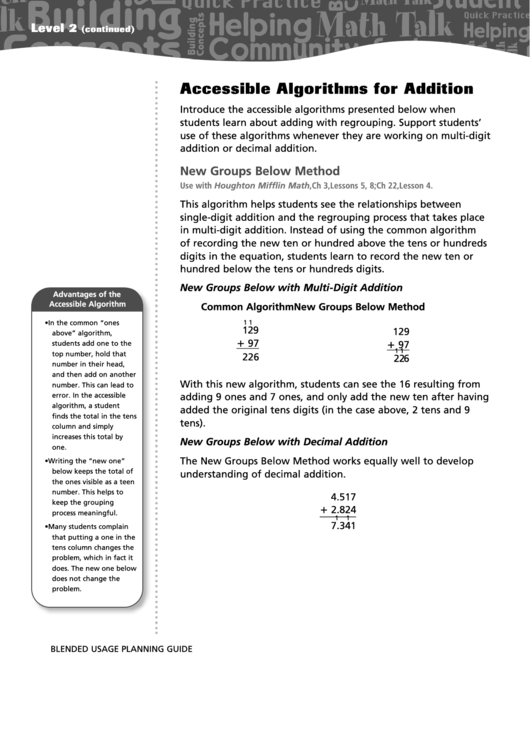
New Groups Below with Multi-Digit Addition
Advantages of the
Accessible Algorithm
Common Algorithm
New Groups Below Method
• In the common “ones
1
1
1
2
9
129
above” algorithm,
97
students add one to the
97
1
1
top number, hold that
226
2
2
6
number in their head,
and then add on another
With this new algorithm, students can see the 16 resulting from
number. This can lead to
error. In the accessible
adding 9 ones and 7 ones, and only add the new ten after having
algorithm, a student
added the original tens digits (in the case above, 2 tens and 9
finds the total in the tens
tens).
column and simply
increases this total by
New Groups Below with Decimal Addition
one.
The New Groups Below Method works equally well to develop
• Writing the “new one”
below keeps the total of
understanding of decimal addition.
the ones visible as a teen
number. This helps to
4.517
keep the grouping
2.824
process meaningful.
1
1
7.
34
1
• Many students complain
that putting a one in the
tens column changes the
problem, which in fact it
does. The new one below
does not change the
problem.
BLENDED USAGE PLANNING GUIDE
ADVERTISEMENT
0 votes
Related Articles
Related forms
Related Categories
Parent category: Education
 1
1 2
2